Dobson Units


DOBSON ident 10-3 cm-amagat
given the number density, N, in molecules per cm3 then
rhox = (<mw>x · Nx(z))/Na grams/cm2
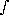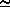C(L) ident integ N(z) dz appeq <N(L)> · Delta z = <N(i)> · Delta P/(rhot g) = q · Na · Delta P/(<mw> g) molecules/cm2
Note that rho = q · rhot = N · <mw> /Na where q is the volumetric mixing ratio of the species being measured.
To convert to mass column density, M, in grams per cm2
M(L) = (<mw>O3 /Na) · C(L) = Delta P/ g
DOBSON = 1000 * Z = 1000 * sumC(L) / N0
where N0 is Loschmidt's number.

The atmosphere consists of fixed gases (e.g., CO2, N2O, CO), water, and ozone so the pressure within any level is given as

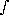
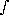Delta p(L) ident integ rhot g dz = g integ (rhof + rhow + rhoo) dz
so that
Delta p(L) · Na / g = <mw>f · Cf(L) + <mw>w · Cw(L) + <mw>o · Co(L)
Cf(L) = (Delta p(L) · Na)/(<mw>f · g) - (<mw>w/<mw>f) · Cw(L) - (<mw>o/<mw>f) · Co(L)
we can also define the total column density as
Ct(L) ident (Delta p(L) · Na) / (<mw>(L) · g)
and if we require
Ct(L) = Cf(L) + Cw(L) + Co(L)
then,
<mw>(L) = (<mw>f · Cf(L) + <mw>w · Cw(L) + <mw>o · Co(L)) / Ct(L)
Ct(L) in eqn 6 and <mw> can be solved for iteratively with an initial guess of <mw> = <mw>f
The volumetric mixing ratio, i.e., molecules of species x to the total number of molecules, is given by
fo(L) = Co(L) / Ct(L) = Co(L) / (Delta P(L) · Na) · <mw>(L) · g