Potential Temperature

Derived directly from integration of the 1st law of thermodynamics. It is the temperature a parcel of air at P and T would have if it were at P_s. It is conserved for adiabatic motions, ( i.e., d/dt = 0).

= T · (P_s/P) = P/( R_g) [(P_s/P)]     = R_g/C_p

For earth = 0.286 (<mw> = 28.96, C_p = 1.004 Joules/gram/K). Some authors write this equation with 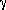= C_p/C_v = 1/(1-)

P/ z = - · g = (P · g)/(R_g · T) = - P/H(z)

log(P) = -H(z) · z
therefore,

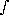P/P_s = e^{- dz'/H(z')}

= T · e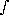^{- dz'/H(z')}
if H(z) = H₀

= T · e^{- · z/H₀}